Informationen
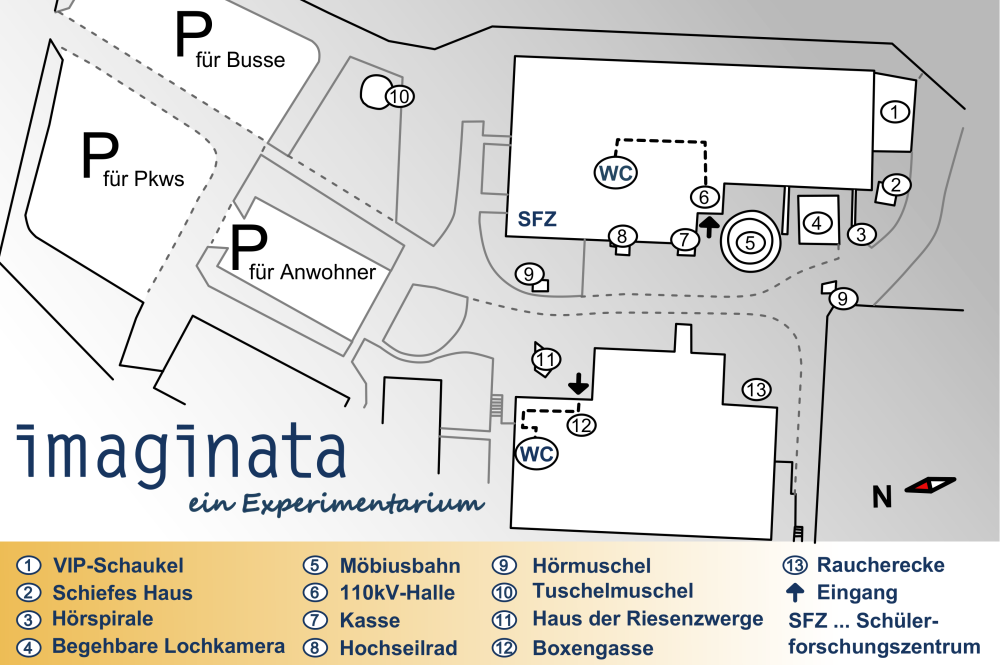
Lageplan
Wir öffnen in 26 Minuten
| Montag - Freitag | 10:00 — 15:00 |
| Samstag - Sonntag | 10:00 — 18:00 |
| 13 Dez - 9 Jan | Geschlossen |
Aktuell gibt es bei uns keine Corona-Beschränkungen.
Wir bitten unsere Gäste dennoch, auf sich und andere Acht zu geben und empfehlen regelmäßige Handhygiene, Abstand halten, sowie das Tragen einer FFP2-Maske.
Stationen
Suche
Außenstationen/Outdoor (2)
Stations-ID: A009
English instruction:
Stand on the platform and hold on to the handrail. Try to get yourself spinning with your feet. What happens if you pull yourself towards the centre?
Stations-ID: A001
?? Work in Progress. English Translation coming soon!
Schreibe einen Kommentar Antworten abbrechen
Innenstationen/Indoor (5)
Stations-ID: D002
?? English instruction:
A normal pendulum swings back and forth Steadily and predictably.
But what happens when you attatch a second pendulum to it?
- What do you think, will both double pendulums move in the same way?
- Hold the longer pendulum and set the short one in motion.
Now let the longer pendulum go. What happens?
Based on his calculations in the field of astronomy, the French physician and mathematician Jules Henri Poincaré (1854-1912) posed the question if all physical phenomena are predictable. In his bestseller “Science and Method” (1912) he gave the following answer: „It is possible, that small differences in the initial circumstances lead to big differences in the results (…) prediction becomes impossible and we are left with a random phenomenon.“
Mehr dazu in unserem Stationenpark-Handbuch
Find out more in our Handbook
Schreibe einen Kommentar Antworten abbrechen
Stations-ID: D091
?? English instruction:
Two people sit across from each other in the carousel, tossing a ball back and forth between them.
The rest of the group sets the carousel in motion. Try tossing the ball back and forth again.
• Where does the ball fly when the carousel is turning?
• Where does the ball fly if the carousel is turning in the opposite direction?
• Which flight path would an observer see, looking at the carousel from the top?
Mehr dazu in unserem Stationenpark-Handbuch
Find out more in our Handbook
Schreibe einen Kommentar Antworten abbrechen
Stations-ID: D006
?? English instruction:
Could the board be transported on these wheels, even though they are not
round?
• How does the axle of the wheels move when they turn?
• Place the board on the wheels.
• Place the board on the axles of the wheels.
• How many corners to a circle?
The rotary piston of the Wankel engine (Mazda MX5) is a type of Reuleaux
wheel.
Mehr dazu in unserem Stationenpark-Handbuch
Find out more in our Handbook
Schreibe einen Kommentar Antworten abbrechen
Stations-ID: D099
English instruction:
Roll bar :
Not just rolls can roll! You can roll all kinds of interesting objects on our roll bar – and experience some surprises in the process! The different objects are presented again on the signs on the side walls. In the middle you will also find a flat table on which you can roll the bodies forward very slowly to observe them closely. Some of the bodies were produced using 3D printing. Can you find out which ones? You can find more information about 3D printing on the board on the right!
Please be very careful with the objects!
Oloids:
Let the two bodies roll down one of tables. Observe which parts of the oloids surface touch the ground!
How do you have to place it at the beginning so that it rolls straight downhill?
The geometry of the oloid is based on two circular disks pushed into each other and rotated by 90°. This body is also called a disk oloid and rolls in a similar fashion as the oloid. The oloid is formed from the disk oloid by connecting the edges of the disks to each other with lateral surfaces.
The initially irritating-looking “antioloid” is based on the same basic geometric shape. If you look a little closer, you will again find the two circles of the disk oloid. Except that in the antioloid they are holes!
You can also recognize similarities to the Möbius strip, however the antioloid has two twists in its “strip” so that there are two clearly defined sides.
Wettrennen/”Downhill racing”:
The two pairs of bodies each have the same weight. Let them each roll down next to each other in pairs. Which of the two bodies is faster? How do they differ from each other?
Bodies with the same weight and external dimensions can still roll at different speeds! This is where rolling differs from falling, because (without air friction) all bodies fall at the same speed.
Although the two cans have the same mass, they contain different liquids. The glicerine in one can is much more viscous than the water in the other. This causes internal friction on the wall of the can, which slows down the rolling motion.
The two bodies with the steel struts are also similar, except for the position of the struts. If the struts are further out, it is more difficult for them to gain momentum because they oppose the rotational movement with a higher moment of inertia.
Sphericons:
The two objects are based on a Sphericon and a Hexasphericon. Place both bodies on the flat table, roll them slowly forward and observe the trajectory. Do you notice a difference between the two bodies?
Sphericons are created when a special body of revolution is cut along the central axis, rotated and rejoined. In the case of the “normal” sphericon, it is a double cone in which one half is rotated by 90° and then rejoined. The resulting body has similarities with an oloid and also rolls similarly. For the hexasphericon, two cones are taken as the basic body, with a cylinder between them. If you cut it in half, you get a regular hexagon as the cut surface. If you now rotate one half by 60 degrees, the cut surfaces fit together again. The resulting body is characterized by a sharp curve in the rolling track.
The bodies here look slightly different because additional cut-outs were made in each case.
The Wobbler:
Take the wobbler and roll it slowly over the flat table. How does it move? Now let it roll down an inclined plane. How do you have to place it so that it rolls straight down?
When rolling, the wobbler looks as if it is moving in serpentine lines. If you were to roll the wheels in paint and let them roll over a sheet of paper, you would actually get a wavy line, a sine curve to be precise! Nevertheless, the center of gravity of the body (similar to the oloid) moves in a straight line downhill.
Incidentally, the wobbler is also quite easy to make yourself! All you need is a roll-shaped piece that you can easily cut up. You could use a salami, for example. Instead of cutting off two straight slices as wheels, simply place the knife at a slight angle. If you now connect the two parts with 3 skewers, for example, you already have a wobbler.
Schreibe einen Kommentar Antworten abbrechen
Stations-ID: D036
?? English instruction:
A long, heavy pendulum is set in motion. Stay a while, calmly observing the patterns it creates.
- Observe the movements of the pendulum, how do they change over time?
- How long does it take until the pendulum rests once more?
- Which basic shape can you recognize in the patterns in the sand?
In 1851, the French physicist Jean Bernard Léon Foucault (1819-1868) proved that the earth rotates using a pendulum of 67m length. In 1997, Imaginata repeated the experiment in the then-gutted ‘Uni-tower’ in Jena, earning a mention in the Guiness book of records. Today, the pendulum draws it’s patterns on the Imaginata’s own premises.
Mehr dazu in unserem Stationenpark-Handbuch
Find out more in our Handbook
Schreibe einen Kommentar Antworten abbrechen
Begleitmaterialien
Interaktiv
Feedback
#imaginata
Problem mit einer Station melden
Bewerte uns!
Es hat dir bei uns gefallen? Du hast Kritik zu äußern? Wir freuen uns über jede faire Bewertung auf Google!




Schreibe einen Kommentar